Difference between revisions of "IC Python API:RLPy RMatrix4"
Chuck (RL) (Talk | contribs) m |
Chuck (RL) (Talk | contribs) m |
||
| (5 intermediate revisions by the same user not shown) | |||
| Line 6: | Line 6: | ||
This class represent the transform data of RTransform. This class provides access to RLPy's internal 4x4 matrix operators and related functions. | This class represent the transform data of RTransform. This class provides access to RLPy's internal 4x4 matrix operators and related functions. | ||
| + | |||
| + | == Constructor & Destructor == | ||
| + | |||
| + | === __init__ ( self, M00 ,M01, M02, M03, M10, M11, M12, M13, M20, M21, M22, M23, M30, M31, M32, M33 ) === | ||
| + | |||
| + | The constructor. Initialize a new [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] with [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] Item Value. | ||
| + | |||
| + | ==== Parameters ==== | ||
| + | :'''M00''' [IN] initialization value - float | ||
| + | :'''M01''' [IN] initialization value - float | ||
| + | :'''M02''' [IN] initialization value - float | ||
| + | :'''M03''' [IN] initialization value - float | ||
| + | :'''M10''' [IN] initialization value - float | ||
| + | :'''M11''' [IN] initialization value - float | ||
| + | :'''M12''' [IN] initialization value - float | ||
| + | :'''M13''' [IN] initialization value - float | ||
| + | :'''M20''' [IN] initialization value - float | ||
| + | :'''M21''' [IN] initialization value - float | ||
| + | :'''M22''' [IN] initialization value - float | ||
| + | :'''M23''' [IN] initialization value - float | ||
| + | :'''M30''' [IN] initialization value - float | ||
| + | :'''M31''' [IN] initialization value - float | ||
| + | :'''M32''' [IN] initialization value - float | ||
| + | :'''M33''' [IN] initialization value - float | ||
| + | |||
| + | ==== Returns ==== | ||
| + | :Returns the row vector of the matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] | ||
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
| + | matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | ||
| + | 5, 6, 7, 8, | ||
| + | 9, 10, 11, 12, | ||
| + | 13, 14, 15, 16 ) | ||
| + | </syntaxhighlight> | ||
| + | |||
| + | === __init__ ( self, Oreder, rx, ty, rz ) === | ||
| + | |||
| + | The constructor. Initialize a new [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] with Order and angle. | ||
| + | |||
| + | ==== Parameters ==== | ||
| + | :'''Oreder''' [IN] Euler order - RLPy.Rotation_Order | ||
| + | :'''rx''' [IN] Angle of x-axis in radians - float | ||
| + | :'''ry''' [IN] Angle of y-axis in radians - float | ||
| + | :'''rz''' [IN] Angle of z-axis in radians - float | ||
| + | |||
| + | ==== Returns ==== | ||
| + | :Returns the row vector of the matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] | ||
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
| + | euler_angle_x = 90 * RLPy.RMath.CONST_DEG_TO_RAD | ||
| + | euler_angle_y = 0 | ||
| + | euler_angle_z = 0 | ||
| + | matrix4 = RLPy.RMatrix4( RLPy.EEulerOrder_XYZ, euler_angle_x, euler_angle_y, euler_angle_z ) | ||
| + | </syntaxhighlight> | ||
| + | |||
| + | === __init__ ( self, rkRotate ) === | ||
| + | |||
| + | The constructor. Initialize a new [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] with [[IC_Python_API:RLPy_RMatrix3|RMatrix3]]. | ||
| + | |||
| + | ==== Parameters ==== | ||
| + | :'''rkRotate''' [IN] Rotation 3x3 matrix - [[IC_Python_API:RLPy_RMatrix3|RMatrix3]] | ||
| + | |||
| + | ==== Returns ==== | ||
| + | :Returns the row vector of the matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] | ||
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
| + | rotate = RLPy.RMatrix3( 1, 0, 0, | ||
| + | 0, 2, 0, | ||
| + | 0, 0, 3 ) | ||
| + | matrix4 = RLPy.RMatrix4( rotate ) | ||
| + | </syntaxhighlight> | ||
| + | |||
| + | === __init__ ( self, kRotate, kTranslate, kScale ) === | ||
| + | |||
| + | The constructor. Initialize a new [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] with RTS. | ||
| + | |||
| + | ==== Parameters ==== | ||
| + | :'''rkRotate''' [IN] Rotation matrix - [[IC_Python_API:RLPy_RMatrix3|RMatrix3]] | ||
| + | :'''rkTranslate''' [IN] Translate vector - [[IC_Python_API:RLPy_RVector3|RVector3]] | ||
| + | :'''rkScale''' [IN] Scale vector - [[IC_Python_API:RLPy_RVector3|RVector3]] | ||
| + | |||
| + | ==== Returns ==== | ||
| + | :Returns the row vector of the matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] | ||
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
| + | rotate = RLPy.RMatrix3( 1, 0, 0, | ||
| + | 0, 2, 0, | ||
| + | 0, 0, 3 ) | ||
| + | translate = RLPy.RVector3( 1,2,3 ) | ||
| + | scale = RLPy.RVector3( 2,2,2 ) | ||
| + | matrix4 = RLPy.RMatrix4( rotate, translate, scale ) | ||
| + | </syntaxhighlight> | ||
| + | |||
| + | === __init__ ( self, args ) === | ||
| + | |||
| + | The constructor. Initialize a new 4x4 matrix object with another [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] object. | ||
| + | |||
| + | ==== Parameters ==== | ||
| + | :'''args''' [IN] a 4x4 matrix object - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] | ||
| + | |||
| + | ==== Returns ==== | ||
| + | :Returns the row vector of the matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] | ||
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
| + | matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | ||
| + | 5, 6, 7, 8, | ||
| + | 9, 10, 11, 12, | ||
| + | 13, 14, 15, 16 ) | ||
| + | matrix4_copy = RLPy.RMatrix4( matrix4 ) | ||
| + | print( matrix4_copy == matrix4 ) # true | ||
| + | </syntaxhighlight> | ||
== Operators == | == Operators == | ||
| Line 13: | Line 124: | ||
The "addition" operator. | The "addition" operator. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#+=|+=]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 34: | Line 147: | ||
The "subtraction" operator. | The "subtraction" operator. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#-=|-=]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 55: | Line 170: | ||
The "multiplication" operator. | The "multiplication" operator. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#*=|*=]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 71: | Line 188: | ||
=== / === | === / === | ||
| − | The "division" operator. | + | The "division" operator. |
| + | |||
| + | See Also: [[#/=|/=]] | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 90: | Line 209: | ||
The "unary minus" . | The "unary minus" . | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 107: | Line 226: | ||
The "equal to" operator. Performs a one-by-one comparison of the matrix array. | The "equal to" operator. Performs a one-by-one comparison of the matrix array. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#!=|!=]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 124: | Line 245: | ||
The "not equal to" operator. Performs a one-by-one comparison of the matrix array. | The "not equal to" operator. Performs a one-by-one comparison of the matrix array. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#==|==]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 141: | Line 264: | ||
The "greater than" operator. Performs a one-by-one comparison of the matrix array. | The "greater than" operator. Performs a one-by-one comparison of the matrix array. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#>=|>=]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4_a = RLPy.RMatrix4( 1, 0, 0, 0, | matrix4_a = RLPy.RMatrix4( 1, 0, 0, 0, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 158: | Line 283: | ||
The "greater than or equal to" operator. Performs a one-by-one comparison of the matrix array. | The "greater than or equal to" operator. Performs a one-by-one comparison of the matrix array. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#>|>]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4_a = RLPy.RMatrix4( 1, 1, 1, 4, | matrix4_a = RLPy.RMatrix4( 1, 1, 1, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 175: | Line 302: | ||
The "less than" operator. Performs a one-by-one comparison of the matrix array. | The "less than" operator. Performs a one-by-one comparison of the matrix array. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#<=|<=]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4_a = RLPy.RMatrix4( 2, 0, 0, 0, | matrix4_a = RLPy.RMatrix4( 2, 0, 0, 0, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 192: | Line 321: | ||
The "less than" operator. Performs a one-by-one comparison of the matrix array. | The "less than" operator. Performs a one-by-one comparison of the matrix array. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#<|<]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4_a = RLPy.RMatrix4( 2, 2, 1, 0, | matrix4_a = RLPy.RMatrix4( 2, 2, 1, 0, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 209: | Line 340: | ||
The "addition assignment" operator. | The "addition assignment" operator. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#+|+]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 229: | Line 362: | ||
The "subtraction assignment" operator. | The "subtraction assignment" operator. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#-|-]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 249: | Line 384: | ||
The "multiplication assignment" operator. For the calculation method, refer to the '''*''' operator. | The "multiplication assignment" operator. For the calculation method, refer to the '''*''' operator. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#*|*]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 266: | Line 403: | ||
The "division assignment" operator. For the calculation method, refer to the '''/''' operator. | The "division assignment" operator. For the calculation method, refer to the '''/''' operator. | ||
| − | <syntaxhighlight lang=" | + | See Also: [[#/|/]] |
| + | |||
| + | <syntaxhighlight lang="python" line='line'> | ||
matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4 = RLPy.RMatrix4( 1, 2, 3, 4, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 285: | Line 424: | ||
This function can be used to initialize the 3x3 matrix. It is equivalent to setting the matrix to: | This function can be used to initialize the 3x3 matrix. It is equivalent to setting the matrix to: | ||
| − | + | [1 0 0 0] | |
| − | + | [0 1 0 0] | |
| − | + | [0 0 1 0] | |
| − | + | [0 0 0 1] | |
==== Returns ==== | ==== Returns ==== | ||
| − | :This object - | + | :This object - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4 = RLPy.RMatrix4() | matrix4 = RLPy.RMatrix4() | ||
matrix4.MakeIdentity() | matrix4.MakeIdentity() | ||
| Line 303: | Line 442: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''nRow'''[IN] Index of the row in the matrix - int'''nCol'''[IN] Index of the column in the matrix - int | + | :'''nRow''' [IN] Index of the row in the matrix - int |
| + | :'''nCol''' [IN] Index of the column in the matrix - int | ||
==== Returns ==== | ==== Returns ==== | ||
:The matrix element specified by row and col - float | :The matrix element specified by row and col - float | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4 = RLPy.RMatrix4() | matrix4 = RLPy.RMatrix4() | ||
matrix4.MakeIdentity() | matrix4.MakeIdentity() | ||
| Line 320: | Line 460: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''nRow'''[IN] Index of the matrix. | + | :'''nRow''' [IN] Index of the matrix. |
==== Returns ==== | ==== Returns ==== | ||
:The matrix element specified by index - float | :The matrix element specified by index - float | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4 = RLPy.RMatrix4() | matrix4 = RLPy.RMatrix4() | ||
matrix4.MakeIdentity() | matrix4.MakeIdentity() | ||
| Line 337: | Line 477: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''nRow'''[IN] Index of the row in the matrix. | + | :'''nRow''' [IN] Index of the row in the matrix. |
==== Returns ==== | ==== Returns ==== | ||
| − | :The row vector of the matrix - | + | :The row vector of the matrix - [[IC_Python_API:RLPy_RVector4|RVector4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4 = RLPy.RMatrix4() | matrix4 = RLPy.RMatrix4() | ||
matrix4.MakeIdentity() | matrix4.MakeIdentity() | ||
| Line 358: | Line 498: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''nRow'''[IN] Index of the column in the matrix. | + | :'''nRow''' [IN] Index of the column in the matrix. |
==== Returns ==== | ==== Returns ==== | ||
| − | :The column vector of the matrix - | + | :The column vector of the matrix - [[IC_Python_API:RLPy_RVector4|RVector4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4 = RLPy.RMatrix4() | matrix4 = RLPy.RMatrix4() | ||
matrix4.MakeIdentity() | matrix4.MakeIdentity() | ||
| Line 379: | Line 519: | ||
==== Returns ==== | ==== Returns ==== | ||
| − | :A new matrix containing this matrix's transpose - | + | :A new matrix containing this matrix's transpose - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_orgin = RLPy.RMatrix4( 1, 2, 3, 4, | ||
5, 6, 7, 8, | 5, 6, 7, 8, | ||
| Line 401: | Line 541: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''mM'''[IN] the matrix - | + | :'''mM''' [IN] the matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
==== Returns ==== | ==== Returns ==== | ||
| − | :A new matrix. (this^T * mM) - | + | :A new matrix. (this^T * mM) - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_orgin = RLPy.RMatrix4( 1, 2, 3, 4, | ||
5, 6, 7, 8, | 5, 6, 7, 8, | ||
| Line 430: | Line 570: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''mM'''[IN] the matrix - | + | :'''mM''' [IN] the matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
==== Returns ==== | ==== Returns ==== | ||
| − | :A new matrix. (this * M^T) - | + | :A new matrix. (this * M^T) - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4( 1, 2, 3, 4, | matrix4_orgin = RLPy.RMatrix4( 1, 2, 3, 4, | ||
5, 6, 7, 8, | 5, 6, 7, 8, | ||
| Line 459: | Line 599: | ||
==== Returns ==== | ==== Returns ==== | ||
| − | :A new matrix containing this matrix's inverse - | + | :A new matrix containing this matrix's inverse - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 480: | Line 620: | ||
==== Returns ==== | ==== Returns ==== | ||
| − | :A new matrix containing this matrix's adjoint - | + | :A new matrix containing this matrix's adjoint - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 501: | Line 641: | ||
==== Returns ==== | ==== Returns ==== | ||
| − | :A new matrix - | + | :A new matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 522: | Line 662: | ||
==== Returns ==== | ==== Returns ==== | ||
| − | :A new matrix - | + | :A new matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 541: | Line 681: | ||
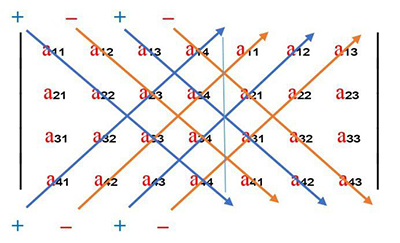
Obtain the scalar value for this 4x4 matrix (|A|). | Obtain the scalar value for this 4x4 matrix (|A|). | ||
| + | |||
| + | [[File:Rlpy_rmatrix4_determinant.jpg]] | ||
==== Returns ==== | ==== Returns ==== | ||
:The determinant of the matrix - float | :The determinant of the matrix - float | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 560: | Line 702: | ||
:Return index of column of M containing maximum abs entry, or -1 if M = 0 - int | :Return index of column of M containing maximum abs entry, or -1 if M = 0 - int | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_column_value = RLPy.RMatrix4( 1, 2, 3,-5, | matrix4_column_value = RLPy.RMatrix4( 1, 2, 3,-5, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 575: | Line 717: | ||
:Return index of row of M containing maximum abs entry, or -1 if M = 0 - int | :Return index of row of M containing maximum abs entry, or -1 if M = 0 - int | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_row_value = RLPy.RMatrix4( 1, 0, 0, 0, | matrix4_row_value = RLPy.RMatrix4( 1, 0, 0, 0, | ||
2, 0, 0, 0, | 2, 0, 0, 0, | ||
| Line 590: | Line 732: | ||
:Return Norm - float | :Return Norm - float | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_row_value = RLPy.RMatrix4( 1, 0, 0, 0, | matrix4_row_value = RLPy.RMatrix4( 1, 0, 0, 0, | ||
2, 0, 0, 0, | 2, 0, 0, 0, | ||
| Line 605: | Line 747: | ||
:Return InfNorm - float | :Return InfNorm - float | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_column_value = RLPy.RMatrix4( 1, 2, 3,-5, | matrix4_column_value = RLPy.RMatrix4( 1, 2, 3,-5, | ||
0, 0, 0, 0, | 0, 0, 0, 0, | ||
| Line 618: | Line 760: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''kRotate '''[IN] Rotate Matrix - | + | :'''kRotate ''' [IN] Rotate Matrix - [[IC_Python_API:RLPy_RMatrix3|RMatrix3]] |
| − | :'''kTranslate'''[IN] Translate vector - | + | :'''kTranslate''' [IN] Translate vector - [[IC_Python_API:RLPy_RVector3|RVector3]] |
| − | :'''kScale '''[IN] Scale vector - | + | :'''kScale ''' [IN] Scale vector - [[IC_Python_API:RLPy_RVector3|RVector3]] |
==== Returns ==== | ==== Returns ==== | ||
| − | :Return a new matrix from RTS - | + | :Return a new matrix from RTS - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
rotate = RLPy.RMatrix3( 1, 0, 0, | rotate = RLPy.RMatrix3( 1, 0, 0, | ||
0, 1, 0, | 0, 1, 0, | ||
| Line 646: | Line 788: | ||
==== Parameters ==== | ==== Parameters ==== | ||
:'''rkRotate''' [IN] Angle of x-axis in radians - float | :'''rkRotate''' [IN] Angle of x-axis in radians - float | ||
| − | :'''rkTranslate'''[IN] Angle of y-axis in radians - float | + | :'''rkTranslate''' [IN] Angle of y-axis in radians - float |
| − | :'''rkScale '''[IN] Angle of z-axis in radians - float | + | :'''rkScale ''' [IN] Angle of z-axis in radians - float |
==== Returns ==== | ==== Returns ==== | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 680: | Line 822: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''rkRotate'''[IN] Rotation Matrix - | + | :'''rkRotate''' [IN] Rotation Matrix - [[IC_Python_API:RLPy_RMatrix3|RMatrix3]] |
==== Returns ==== | ==== Returns ==== | ||
| − | : | + | :3x3 matrix rotation data of this 4x4 matrix. |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 696: | Line 838: | ||
print(row0[0]) | print(row0[0]) | ||
print(row0[1]) | print(row0[1]) | ||
| − | print(row0[2]) | + | print(row0[2]) |
</syntaxhighlight> | </syntaxhighlight> | ||
| Line 703: | Line 845: | ||
Set the translation data in this 4x4 matrix to 0. | Set the translation data in this 4x4 matrix to 0. | ||
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 718: | Line 860: | ||
=== RotationX (self, fAngle) === | === RotationX (self, fAngle) === | ||
| − | Rotation matrix for rotations around x- | + | Rotation matrix for rotations around x-axis. |
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''fAngle'''[IN] angle in radians - float | + | :'''fAngle''' [IN] angle in radians - float |
==== Returns ==== | ==== Returns ==== | ||
| − | :Return a new matrix of for rotations around x-axis - | + | :Return a new matrix of for rotations around x-axis - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4() | matrix4_orgin = RLPy.RMatrix4() | ||
matrix4_orgin.MakeIdentity() | matrix4_orgin.MakeIdentity() | ||
| Line 737: | Line 879: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''fAngle'''[IN] angle in radians - float | + | :'''fAngle''' [IN] angle in radians - float |
==== Returns ==== | ==== Returns ==== | ||
| − | :Return a new matrix of for rotations around y-axis - | + | :Return a new matrix of for rotations around y-axis - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4() | matrix4_orgin = RLPy.RMatrix4() | ||
matrix4_orgin.MakeIdentity() | matrix4_orgin.MakeIdentity() | ||
| Line 753: | Line 895: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''fAngle'''[IN] angle in radians - float | + | :'''fAngle''' [IN] angle in radians - float |
==== Returns ==== | ==== Returns ==== | ||
| − | :Return a new matrix of for rotations around z-axis - | + | :Return a new matrix of for rotations around z-axis - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4() | matrix4_orgin = RLPy.RMatrix4() | ||
matrix4_orgin.MakeIdentity() | matrix4_orgin.MakeIdentity() | ||
| Line 769: | Line 911: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''rkAxis'''[IN] axis vector - | + | :'''rkAxis''' [IN] axis vector - [[IC_Python_API:RLPy_RVector3|RVector3]] |
| − | :'''fAngle'''[IN] angle in radians - float | + | :'''fAngle''' [IN] angle in radians - float |
==== Returns ==== | ==== Returns ==== | ||
| − | :Return a new matrix from specified axis angle - | + | :Return a new matrix from specified axis angle - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 793: | Line 935: | ||
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''Oreder'''[IN] Euler order - | + | :'''Oreder''' [IN] Euler order - RLPY.EEulerOrder |
| + | :*EEulerOrder_XYZ = _RLPy.EEulerOrder_XYZ | ||
| + | :*EEulerOrder_ZYX = _RLPy.EEulerOrder_ZYX | ||
| + | :*EEulerOrder_XZY = _RLPy.EEulerOrder_XZY | ||
| + | :*EEulerOrder_YZX = _RLPy.EEulerOrder_YZX | ||
| + | :*EEulerOrder_YXZ = _RLPy.EEulerOrder_YXZ | ||
| + | :*EEulerOrder_ZXY = _RLPy.EEulerOrder_ZXY | ||
| + | :'''rx''' [IN] Angle of x-axis in radians - float | ||
| + | :'''ry''' [IN] Angle of y-axis in radians - float | ||
| + | :'''rz''' [IN] Angle of z-axis in radians - float | ||
==== Returns ==== | ==== Returns ==== | ||
| − | :Return a new matrix from specified axis angle - | + | :Return a new matrix from specified axis angle - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
euler_angle_x = 90 * RLPy.RMath.CONST_DEG_TO_RAD | euler_angle_x = 90 * RLPy.RMath.CONST_DEG_TO_RAD | ||
euler_angle_y = 0 | euler_angle_y = 0 | ||
| Line 813: | Line 964: | ||
=== SetSR (self, mSR) === | === SetSR (self, mSR) === | ||
| − | Set scale and rotation part of the | + | Set scale and rotation part of the matrix. |
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''mSR'''[IN] 3x3 matrix - | + | :'''mSR''' [IN] 3x3 matrix - [[IC_Python_API:RLPy_RMatrix3|RMatrix3]] |
==== Returns ==== | ==== Returns ==== | ||
| − | :Return a new 4x4 matrix - | + | :Return a new 4x4 matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4() | matrix4_orgin = RLPy.RMatrix4() | ||
matrix4_orgin.MakeIdentity() | matrix4_orgin.MakeIdentity() | ||
| Line 832: | Line 983: | ||
=== GetSR (self) === | === GetSR (self) === | ||
| − | Get scale and rotation part of the | + | Get scale and rotation part of the matrix. |
==== Returns ==== | ==== Returns ==== | ||
| − | :Return a 3x3 matrix - | + | :Return a 3x3 matrix - [[IC_Python_API:RLPy_RMatrix3|RMatrix3]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1, | ||
1, 1,-1,-2, | 1, 1,-1,-2, | ||
| Line 851: | Line 1,002: | ||
=== SetTranslate (self, vTranslate) === | === SetTranslate (self, vTranslate) === | ||
| − | Set translate of the | + | Set translate of the matrix. |
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''vTranslate'''[IN] Translate vector - | + | :'''vTranslate''' [IN] Translate vector - [[IC_Python_API:RLPy_RVector3|RVector3]] |
==== Returns ==== | ==== Returns ==== | ||
| − | : | + | :New matrix with the specified translation - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4() | matrix4_orgin = RLPy.RMatrix4() | ||
matrix4_orgin.MakeIdentity() | matrix4_orgin.MakeIdentity() | ||
| Line 867: | Line 1,018: | ||
=== GetTranslate (self) === | === GetTranslate (self) === | ||
| − | Get translate of the | + | Get translate of the matrix. |
==== Returns ==== | ==== Returns ==== | ||
| − | :Return a translate vector - | + | :Return a translate vector - [[IC_Python_API:RLPy_RVector3|RVector3]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4() | matrix4_orgin = RLPy.RMatrix4() | ||
matrix4_orgin.MakeIdentity() | matrix4_orgin.MakeIdentity() | ||
| Line 885: | Line 1,036: | ||
=== AccuScale (self, rkScale) === | === AccuScale (self, rkScale) === | ||
| − | Accumulate matrix with scale | + | Accumulate this 4x4 matrix with scale vector. |
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''rkScale'''[IN] Scale vector - | + | :'''rkScale''' [IN] Scale vector - [[IC_Python_API:RLPy_RVector3|RVector3]] |
==== Returns ==== | ==== Returns ==== | ||
| − | : | + | :Accumulate of this 4x4 matrix with scale vector - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4() | matrix4_orgin = RLPy.RMatrix4() | ||
matrix4_orgin.MakeIdentity() | matrix4_orgin.MakeIdentity() | ||
| Line 909: | Line 1,060: | ||
=== AccuRotate (self, rkRotate) === | === AccuRotate (self, rkRotate) === | ||
| − | Accumulate matrix with rotation | + | Accumulate this 4x4 matrix with rotation matrix. |
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''rkRotate'''[IN] Rotation matrix - | + | :'''rkRotate''' [IN] Rotation matrix - [[IC_Python_API:RLPy_RMatrix3|RMatrix3]] |
==== Returns ==== | ==== Returns ==== | ||
| − | : | + | :Accumulate this 4x4 matrix and rotation matrix - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4() | matrix4_orgin = RLPy.RMatrix4() | ||
matrix4_orgin.MakeIdentity() | matrix4_orgin.MakeIdentity() | ||
| Line 934: | Line 1,085: | ||
=== AccuTranslate (self, rkTranslate) === | === AccuTranslate (self, rkTranslate) === | ||
| − | Accumulate matrix with translate | + | Accumulate this 4x4 matrix with translate vector. |
==== Parameters ==== | ==== Parameters ==== | ||
| − | :'''rkTranslate'''[IN] Translate vector - | + | :'''rkTranslate''' [IN] Translate vector - [[IC_Python_API:RLPy_RVector3|RVector3]] |
==== Returns ==== | ==== Returns ==== | ||
| − | : | + | :Accumulate of this 4x4 matrix and translation vector - [[IC_Python_API:RLPy_RMatrix4|RMatrix4]] |
| − | <syntaxhighlight lang=" | + | <syntaxhighlight lang="python" line='line'> |
matrix4_orgin = RLPy.RMatrix4() | matrix4_orgin = RLPy.RMatrix4() | ||
matrix4_orgin.MakeIdentity() | matrix4_orgin.MakeIdentity() | ||
Latest revision as of 23:48, 14 April 2020
Contents
- 1 Description
- 2 Constructor & Destructor
- 3 Operators
- 4 Member Functions
- 4.1 MakeIdentity (self)
- 4.2 M (self, args)
- 4.3 E (self, args)
- 4.4 GetRow (self, nR)
- 4.5 GetColumn (self, nC)
- 4.6 Transpose (self)
- 4.7 TransposeTimes (self, mM)
- 4.8 TimesTranspose (self, mM)
- 4.9 Inverse (self)
- 4.10 Adjoint (self)
- 4.11 AdjointTranspose (self)
- 4.12 InverseTranspose (self)
- 4.13 Determinant (self)
- 4.14 MaxColumn (self)
- 4.15 MaxRow (self)
- 4.16 OneNorm (self)
- 4.17 InfNorm (self)
- 4.18 FromRTS (self, kRotate, kTranslate, kScale)
- 4.19 GetSimpleRTS (self, rkRotate, rkTranslate, rkScale)
- 4.20 GetSimpleRotate (self, rkRotate)
- 4.21 SetTranslateZero (self)
- 4.22 RotationX (self, fAngle)
- 4.23 RotationY (self, fAngle)
- 4.24 RotationZ (self, fAngle)
- 4.25 RotateAxisAngle (self, rkAxis, fAngle)
- 4.26 FromEulerAngle (self, Oreder, rx, ry, rz)
- 4.27 SetSR (self, mSR)
- 4.28 GetSR (self)
- 4.29 SetTranslate (self, vTranslate)
- 4.30 GetTranslate (self)
- 4.31 AccuScale (self, rkScale)
- 4.32 AccuRotate (self, rkRotate)
- 4.33 AccuTranslate (self, rkTranslate)
- Main article: Modules.
- Last modified: 04/14/2020
Description
This class represent the transform data of RTransform. This class provides access to RLPy's internal 4x4 matrix operators and related functions.
Constructor & Destructor
__init__ ( self, M00 ,M01, M02, M03, M10, M11, M12, M13, M20, M21, M22, M23, M30, M31, M32, M33 )
The constructor. Initialize a new RMatrix4 with RMatrix4 Item Value.
Parameters
- M00 [IN] initialization value - float
- M01 [IN] initialization value - float
- M02 [IN] initialization value - float
- M03 [IN] initialization value - float
- M10 [IN] initialization value - float
- M11 [IN] initialization value - float
- M12 [IN] initialization value - float
- M13 [IN] initialization value - float
- M20 [IN] initialization value - float
- M21 [IN] initialization value - float
- M22 [IN] initialization value - float
- M23 [IN] initialization value - float
- M30 [IN] initialization value - float
- M31 [IN] initialization value - float
- M32 [IN] initialization value - float
- M33 [IN] initialization value - float
Returns
- Returns the row vector of the matrix - RMatrix4
1 matrix4 = RLPy.RMatrix4( 1, 2, 3, 4,
2 5, 6, 7, 8,
3 9, 10, 11, 12,
4 13, 14, 15, 16 )
__init__ ( self, Oreder, rx, ty, rz )
The constructor. Initialize a new RMatrix4 with Order and angle.
Parameters
- Oreder [IN] Euler order - RLPy.Rotation_Order
- rx [IN] Angle of x-axis in radians - float
- ry [IN] Angle of y-axis in radians - float
- rz [IN] Angle of z-axis in radians - float
Returns
- Returns the row vector of the matrix - RMatrix4
1 euler_angle_x = 90 * RLPy.RMath.CONST_DEG_TO_RAD
2 euler_angle_y = 0
3 euler_angle_z = 0
4 matrix4 = RLPy.RMatrix4( RLPy.EEulerOrder_XYZ, euler_angle_x, euler_angle_y, euler_angle_z )
__init__ ( self, rkRotate )
The constructor. Initialize a new RMatrix4 with RMatrix3.
Parameters
- rkRotate [IN] Rotation 3x3 matrix - RMatrix3
Returns
- Returns the row vector of the matrix - RMatrix4
1 rotate = RLPy.RMatrix3( 1, 0, 0,
2 0, 2, 0,
3 0, 0, 3 )
4 matrix4 = RLPy.RMatrix4( rotate )
__init__ ( self, kRotate, kTranslate, kScale )
The constructor. Initialize a new RMatrix4 with RTS.
Parameters
- rkRotate [IN] Rotation matrix - RMatrix3
- rkTranslate [IN] Translate vector - RVector3
- rkScale [IN] Scale vector - RVector3
Returns
- Returns the row vector of the matrix - RMatrix4
1 rotate = RLPy.RMatrix3( 1, 0, 0,
2 0, 2, 0,
3 0, 0, 3 )
4 translate = RLPy.RVector3( 1,2,3 )
5 scale = RLPy.RVector3( 2,2,2 )
6 matrix4 = RLPy.RMatrix4( rotate, translate, scale )
__init__ ( self, args )
The constructor. Initialize a new 4x4 matrix object with another RMatrix4 object.
Parameters
- args [IN] a 4x4 matrix object - RMatrix4
Returns
- Returns the row vector of the matrix - RMatrix4
1 matrix4 = RLPy.RMatrix4( 1, 2, 3, 4,
2 5, 6, 7, 8,
3 9, 10, 11, 12,
4 13, 14, 15, 16 )
5 matrix4_copy = RLPy.RMatrix4( matrix4 )
6 print( matrix4_copy == matrix4 ) # true
Operators
+
The "addition" operator.
See Also: +=
1 matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_b = RLPy.RMatrix4( 2, 2, 2, 2,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9 matrix4_result = matrix4_a + matrix4_b
10
11 print( matrix4_result.GetRow(0)[0] == 1+2 ) # true
12 print( matrix4_result.GetRow(0)[1] == 2+2 ) # true
13 print( matrix4_result.GetRow(0)[2] == 3+2 ) # true
14 print( matrix4_result.GetRow(0)[3] == 4+2 ) # true
-
The "subtraction" operator.
See Also: -=
1 matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_b = RLPy.RMatrix4( 2, 2, 2, 2,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9 matrix4_result = matrix4_a - matrix4_b
10
11 print( matrix4_result.GetRow(0)[0] == 1-2 ) # true
12 print( matrix4_result.GetRow(0)[1] == 2-2 ) # true
13 print( matrix4_result.GetRow(0)[2] == 3-2 ) # true
14 print( matrix4_result.GetRow(0)[3] == 4-2 ) # true
*
The "multiplication" operator.
See Also: *=
1 matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_b = RLPy.RMatrix4( 2, 0, 0, 0,
6 2, 0, 0, 0,
7 2, 0, 0, 0,
8 2, 0, 0, 0 )
9 matrix4_result = matrix4_a * matrix4_b
10
11 print( matrix4_result.GetRow(0)[0] == 1*2 + 2*2 + 3*2 + 4*2 ) # true
/
The "division" operator.
See Also: /=
1 matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_result = matrix4_a / 2
6
7 print( matrix4_result.GetRow(0)[0] == 1/2 ) # true
8 print( matrix4_result.GetRow(0)[1] == 2/2 ) # true
9 print( matrix4_result.GetRow(0)[2] == 3/2 ) # true
10 print( matrix4_result.GetRow(0)[3] == 4/2 ) # true
-
The "unary minus" .
1 matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_result = -matrix4_a
6
7 print( matrix4_result.GetRow(0)[0] == -1 ) # true
8 print( matrix4_result.GetRow(0)[1] == -2 ) # true
9 print( matrix4_result.GetRow(0)[2] == -3 ) # true
10 print( matrix4_result.GetRow(0)[3] == -4 ) # true
==
The "equal to" operator. Performs a one-by-one comparison of the matrix array.
See Also: !=
1 matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_b = RLPy.RMatrix4( 1, 2, 3, 4,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9
10 print( matrix4_a == matrix4_b ) # true
!=
The "not equal to" operator. Performs a one-by-one comparison of the matrix array.
See Also: ==
1 matrix4_a = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_b = RLPy.RMatrix4( 2, 2, 2, 2,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9
10 print( matrix4_a != matrix4_b ) # true
>
The "greater than" operator. Performs a one-by-one comparison of the matrix array.
See Also: >=
1 matrix4_a = RLPy.RMatrix4( 1, 0, 0, 0,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_b = RLPy.RMatrix4( 2, 0, 0, 0,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9
10 print( matrix4_b > matrix4_a ) # true
>=
The "greater than or equal to" operator. Performs a one-by-one comparison of the matrix array.
See Also: >
1 matrix4_a = RLPy.RMatrix4( 1, 1, 1, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_b = RLPy.RMatrix4( 1, 1, 1, 8,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9
10 print( matrix4_b >= matrix4_a ) # true
<
The "less than" operator. Performs a one-by-one comparison of the matrix array.
See Also: <=
1 matrix4_a = RLPy.RMatrix4( 2, 0, 0, 0,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_b = RLPy.RMatrix4( 3, 0, 0, 0,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9
10 print( matrix4_a < matrix4_b ) # true
<=
The "less than" operator. Performs a one-by-one comparison of the matrix array.
See Also: <
1 matrix4_a = RLPy.RMatrix4( 2, 2, 1, 0,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4_b = RLPy.RMatrix4( 2, 2, 5, 0,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9
10 print( matrix4_a <= matrix4_b ) # true
+=
The "addition assignment" operator.
See Also: +
1 matrix4 = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4 += RLPy.RMatrix4( 2, 2, 2, 2,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9
10 print( matrix4.GetRow(0)[0] == 1+2 ) # true
11 print( matrix4.GetRow(0)[1] == 2+2 ) # true
12 print( matrix4.GetRow(0)[2] == 3+2 ) # true
13 print( matrix4.GetRow(0)[3] == 4+2 ) # true
-=
The "subtraction assignment" operator.
See Also: -
1 matrix4 = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4 -= RLPy.RMatrix4( 2, 2, 2, 2,
6 0, 0, 0, 0,
7 0, 0, 0, 0,
8 0, 0, 0, 0 )
9
10 print( matrix4.GetRow(0)[0] == 1-2 ) # true
11 print( matrix4.GetRow(0)[1] == 2-2 ) # true
12 print( matrix4.GetRow(0)[2] == 3-2 ) # true
13 print( matrix4.GetRow(0)[3] == 4-2 ) # true
*=
The "multiplication assignment" operator. For the calculation method, refer to the * operator.
See Also: *
1 matrix4 = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4 *= 2
6
7 print( matrix4.GetRow(0)[0] == 1*2 ) # true
8 print( matrix4.GetRow(0)[1] == 2*2 ) # true
9 print( matrix4.GetRow(0)[2] == 3*2 ) # true
10 print( matrix4.GetRow(0)[3] == 4*2 ) # true
/=
The "division assignment" operator. For the calculation method, refer to the / operator.
See Also: /
1 matrix4 = RLPy.RMatrix4( 1, 2, 3, 4,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 matrix4 /= 2
6
7 print( matrix4.GetRow(0)[0] == 1/2 ) # true
8 print( matrix4.GetRow(0)[1] == 2/2 ) # true
9 print( matrix4.GetRow(0)[2] == 3/2 ) # true
10 print( matrix4.GetRow(0)[3] == 4/2 ) # true
Member Functions
MakeIdentity (self)
This function can be used to initialize the 3x3 matrix. It is equivalent to setting the matrix to:
[1 0 0 0] [0 1 0 0] [0 0 1 0] [0 0 0 1]
Returns
- This object - RMatrix4
1 matrix4 = RLPy.RMatrix4()
2 matrix4.MakeIdentity()
M (self, args)
Get the value of an element in a 4x4 matrix by row and column index.
Parameters
- nRow [IN] Index of the row in the matrix - int
- nCol [IN] Index of the column in the matrix - int
Returns
- The matrix element specified by row and col - float
1 matrix4 = RLPy.RMatrix4()
2 matrix4.MakeIdentity()
3
4 print(matrix4.M(0,0)) #
E (self, args)
Get the value of an element in a 3x3 matrix by index number (from 0 to 15);
Parameters
- nRow [IN] Index of the matrix.
Returns
- The matrix element specified by index - float
1 matrix4 = RLPy.RMatrix4()
2 matrix4.MakeIdentity()
3
4 print(matrix4.E(0)) #
GetRow (self, nR)
Retreive a row inside a 4x4 matrix.
Parameters
- nRow [IN] Index of the row in the matrix.
Returns
- The row vector of the matrix - RVector4
1 matrix4 = RLPy.RMatrix4()
2 matrix4.MakeIdentity()
3 row0 = matrix4.GetRow(0)
4
5 print(row0[0])
6 print(row0[1])
7 print(row0[2])
8 print(row0[3])
GetColumn (self, nC)
Retrieve a column inside a 4x4 matrix.
Parameters
- nRow [IN] Index of the column in the matrix.
Returns
- The column vector of the matrix - RVector4
1 matrix4 = RLPy.RMatrix4()
2 matrix4.MakeIdentity()
3 col0 = matrix4.GetColumn(0)
4
5 print(col0[0])
6 print(col0[1])
7 print(col0[2])
8 print(col0[3])
Transpose (self)
Obtain the transposed matrix by transposing the current m * n matrix into an n * m matrix by row-column swapping.
Returns
- A new matrix containing this matrix's transpose - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4( 1, 2, 3, 4,
2 5, 6, 7, 8,
3 9, 10, 11, 12,
4 13, 14, 15, 16 )
5 matrix4_transpose = matrix4_orgin.Transpose()
6 row0 = matrix4_orgin.GetRow(0)
7 col0 = matrix4_transpose.GetColumn(0)
8
9 print(row0[0] == col0[0])
10 print(row0[1] == col0[1])
11 print(row0[2] == col0[2])
12 print(row0[3] == col0[3])
TransposeTimes (self, mM)
Multiply a transposed version of a 4x4 matrix with itself.
Parameters
- mM [IN] the matrix - RMatrix4
Returns
- A new matrix. (this^T * mM) - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4( 1, 2, 3, 4,
2 5, 6, 7, 8,
3 9, 10, 11, 12,
4 13, 14, 15, 16 )
5 matrix4_transpose_value = RLPy.RMatrix4( 2, 0, 0, 0,
6 0, 2, 0, 0,
7 0, 0, 2, 0,
8 0, 0, 0, 2 )
9 matrix4_transpose_times = matrix4_orgin.TransposeTimes(matrix4_transpose_value)
10 row0 = matrix4_orgin.GetRow(0)
11 col0 = matrix4_transpose_times.GetColumn(0)
12
13 print(row0[0]*2 == col0[0])
14 print(row0[1]*2 == col0[1])
15 print(row0[2]*2 == col0[2])
16 print(row0[3]*2 == col0[3])
TimesTranspose (self, mM)
Multiply this 4x4 matrix with a transposed version of itself.
Parameters
- mM [IN] the matrix - RMatrix4
Returns
- A new matrix. (this * M^T) - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4( 1, 2, 3, 4,
2 5, 6, 7, 8,
3 9, 10, 11, 12,
4 13, 14, 15, 16 )
5 matrix4_transpose_value = RLPy.RMatrix4( 3, 0, 0, 0,
6 0, 3, 0, 0,
7 0, 0, 3, 0,
8 0, 0, 0, 3 )
9 matrix4_times_transpose = matrix4_orgin.TimesTranspose(matrix4_transpose_value)
10 row0 = matrix4_orgin.GetColumn(0)
11 col0 = matrix4_times_transpose.GetColumn(0)
12
13 print(row0[0]*3 == col0[0])
14 print(row0[1]*3 == col0[1])
15 print(row0[2]*3 == col0[2])
16 print(row0[3]*3 == col0[3])
Inverse (self)
Obtain the inverse (reciprocal) of this 4x4 matrix (A^-1).
Returns
- A new matrix containing this matrix's inverse - RMatrix4
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1 )
5 matrix4_inverse = matrix4_value.Inverse()
6 row0_inverse = matrix4_inverse.GetRow(0)
7
8 print(row0_inverse[0])
9 print(row0_inverse[1])
10 print(row0_inverse[2])
11 print(row0_inverse[3])
Adjoint (self)
Adjugate this 4x4 matrix.
Returns
- A new matrix containing this matrix's adjoint - RMatrix4
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1 )
5 matrix4_Adjoint = matrix4_value.Adjoint()
6 row0_Adjoint = matrix4_Adjoint.GetRow(0)
7
8 print(row0_Adjoint[0])
9 print(row0_Adjoint[1])
10 print(row0_Adjoint[2])
11 print(row0_Adjoint[3])
AdjointTranspose (self)
Adjugate and transpose this 4x4 matrix.
Returns
- A new matrix - RMatrix4
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1 )
5 matrix4_Adjoint_transpose = matrix4_value.AdjointTranspose()
6 col0_Adjoint_transpose = matrix4_Adjoint_transpose.GetColumn(0)
7
8 print(col0_Adjoint_transpose[0] == row0_Adjoint[0])
9 print(col0_Adjoint_transpose[1] == row0_Adjoint[1])
10 print(col0_Adjoint_transpose[2] == row0_Adjoint[2])
11 print(col0_Adjoint_transpose[3] == row0_Adjoint[3])
InverseTranspose (self)
Invert and transpose this 4x4 matrix.
Returns
- A new matrix - RMatrix4
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1 )
5 matrix4_inverse_transpose = matrix4_value.InverseTranspose()
6 col0_inverse_transpose = matrix4_inverse_transpose.GetColumn(0)
7
8 print(col0_inverse_transpose[0] == row0_inverse[0])
9 print(col0_inverse_transpose[1] == row0_inverse[1])
10 print(col0_inverse_transpose[2] == row0_inverse[2])
11 print(col0_inverse_transpose[3] == row0_inverse[3])
Determinant (self)
Obtain the scalar value for this 4x4 matrix (|A|).
Returns
- The determinant of the matrix - float
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1 )
5 print(matrix4_value.Determinant())
MaxColumn (self)
Find the maximum absolute value within this 4x4 matrix, and return the column in which the value is located. If all of the elements within the 4x4 matrix are 0 then return -1.
Returns
- Return index of column of M containing maximum abs entry, or -1 if M = 0 - int
1 matrix4_column_value = RLPy.RMatrix4( 1, 2, 3,-5,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 print(matrix4_column_value.MaxColumn()) # column:3 -> abs(-5)
MaxRow (self)
Find the maximum absolute value within this 4x4 matrix, and return the row in which the value is located. If all of the elements within the 4x4 matrix are 0 then return -1.
Returns
- Return index of row of M containing maximum abs entry, or -1 if M = 0 - int
1 matrix4_row_value = RLPy.RMatrix4( 1, 0, 0, 0,
2 2, 0, 0, 0,
3 3, 0, 0, 0,
4 -5, 0, 0, 0 )
5 print(matrix4_value.MaxRow()) # Row:3 -> abs(-5)
OneNorm (self)
Return the sum of the column elements that contain the largest absolute values.
Returns
- Return Norm - float
1 matrix4_row_value = RLPy.RMatrix4( 1, 0, 0, 0,
2 2, 0, 0, 0,
3 3, 0, 0, 0,
4 -5, 0, 0, 0 )
5 print(matrix4_row_value.OneNorm()) # 11 -> 1+2+abs(-5)
InfNorm (self)
Return the sum of the row elements that contain the largest absolute values.
Returns
- Return InfNorm - float
1 matrix4_column_value = RLPy.RMatrix4( 1, 2, 3,-5,
2 0, 0, 0, 0,
3 0, 0, 0, 0,
4 0, 0, 0, 0 )
5 print(matrix4_column_value.InfNorm()) # 11 -> 1+2+abs(-5)
FromRTS (self, kRotate, kTranslate, kScale)
Apply rotate, translate, and scale data to a 4x4 matrix.
Parameters
- kRotate [IN] Rotate Matrix - RMatrix3
- kTranslate [IN] Translate vector - RVector3
- kScale [IN] Scale vector - RVector3
Returns
- Return a new matrix from RTS - RMatrix4
1 rotate = RLPy.RMatrix3( 1, 0, 0,
2 0, 1, 0,
3 0, 0, 1 )
4 translate = RLPy.RVector3( 1, 0, 0 )
5 scale = RLPy.RVector3( 2, 2, 2 )
6 matrix4_result = RLPy.RMatrix4().FromRTS( rotate, translate, scale )
7 row0 = matrix4_result.GetRow(0)
8
9 print(row0[0])
10 print(row0[1])
11 print(row0[2])
12 print(row0[3])
GetSimpleRTS (self, rkRotate, rkTranslate, rkScale)
Retrieve rotation, translation, and scale data from this 4x4 matrix.
Parameters
- rkRotate [IN] Angle of x-axis in radians - float
- rkTranslate [IN] Angle of y-axis in radians - float
- rkScale [IN] Angle of z-axis in radians - float
Returns
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1 )
5 rotate = RLPy.RMatrix3()
6 translate = RLPy.RVector3()
7 scale = RLPy.RVector3()
8 matrix4_value.GetSimpleRTS( rotate, translate, scale )
9 row0 = rotate.GetRow(0)
10
11 print(row0[0])
12 print(row0[1])
13 print(row0[2])
14
15 print(translate[0])
16 print(translate[1])
17 print(translate[2])
18
19 print(scale[0])
20 print(scale[1])
21 print(scale[2])
GetSimpleRotate (self, rkRotate)
Retrieve rotation data from this 4x4 matrix.
Parameters
- rkRotate [IN] Rotation Matrix - RMatrix3
Returns
- 3x3 matrix rotation data of this 4x4 matrix.
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1
5 rotate = RLPy.RMatrix3()
6 matrix4_value.GetSimpleRotate( rotate )
7 row0 = rotate.GetRow(0)
8
9 print(row0[0])
10 print(row0[1])
11 print(row0[2])
SetTranslateZero (self)
Set the translation data in this 4x4 matrix to 0.
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1
5 matrix4_value.SetTranslateZero()
6 row3 = matrix4_value.GetRow(3)
7
8 print(row3[0] == 0)
9 print(row3[1] == 0)
10 print(row3[2] == 0)
RotationX (self, fAngle)
Rotation matrix for rotations around x-axis.
Parameters
- fAngle [IN] angle in radians - float
Returns
- Return a new matrix of for rotations around x-axis - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4()
2 matrix4_orgin.MakeIdentity()
3 matrix4_orgin.RotationX( 90 * RLPy.RMath.CONST_DEG_TO_RAD )
RotationY (self, fAngle)
Rotation matrix for rotations around y-axis.
Parameters
- fAngle [IN] angle in radians - float
Returns
- Return a new matrix of for rotations around y-axis - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4()
2 matrix4_orgin.MakeIdentity()
3 matrix4_orgin.RotationY( 90 * RLPy.RMath.CONST_DEG_TO_RAD )
RotationZ (self, fAngle)
Rotation matrix for rotations around z-axis.
Parameters
- fAngle [IN] angle in radians - float
Returns
- Return a new matrix of for rotations around z-axis - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4()
2 matrix4_orgin.MakeIdentity()
3 matrix4_orgin.RotationZ( 90 * RLPy.RMath.CONST_DEG_TO_RAD )
RotateAxisAngle (self, rkAxis, fAngle)
Rotation matrix from axis angle.
Parameters
- rkAxis [IN] axis vector - RVector3
- fAngle [IN] angle in radians - float
Returns
- Return a new matrix from specified axis angle - RMatrix4
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1
5 x_axis_vector = RLPy.RVector3( 1, 0, 0 ) # axis = "X"
6 y_axis_vector = RLPy.RVector3( 0, 1, 0 ) # axis = "Y"
7 z_axis_vector = RLPy.RVector3( 0, 0, 1 ) # axis = "Z"
8 matrix4_value.RotateAxisAngle( x_axis_vector, 90 * RLPy.RMath.CONST_DEG_TO_RAD )
9 matrix4_value.RotateAxisAngle( y_axis_vector, 90 * RLPy.RMath.CONST_DEG_TO_RAD )
10 matrix4_value.RotateAxisAngle( z_axis_vector, 90 * RLPy.RMath.CONST_DEG_TO_RAD )
FromEulerAngle (self, Oreder, rx, ry, rz)
Convert Euler angle to a 4x4 matrix according to a rotation axis order.
Parameters
- Oreder [IN] Euler order - RLPY.EEulerOrder
- EEulerOrder_XYZ = _RLPy.EEulerOrder_XYZ
- EEulerOrder_ZYX = _RLPy.EEulerOrder_ZYX
- EEulerOrder_XZY = _RLPy.EEulerOrder_XZY
- EEulerOrder_YZX = _RLPy.EEulerOrder_YZX
- EEulerOrder_YXZ = _RLPy.EEulerOrder_YXZ
- EEulerOrder_ZXY = _RLPy.EEulerOrder_ZXY
- rx [IN] Angle of x-axis in radians - float
- ry [IN] Angle of y-axis in radians - float
- rz [IN] Angle of z-axis in radians - float
Returns
- Return a new matrix from specified axis angle - RMatrix4
1 euler_angle_x = 90 * RLPy.RMath.CONST_DEG_TO_RAD
2 euler_angle_y = 0
3 euler_angle_z = 0
4 matrix4_result = RLPy.RMatrix4().FromEulerAngle( RLPy.EEulerOrder_XYZ, euler_angle_x, euler_angle_y, euler_angle_z)
5 row0 = matrix4_result[0].GetRow(0)
6
7 print(row0[0])
8 print(row0[1])
9 print(row0[2])
10 print(row0[3])
SetSR (self, mSR)
Set scale and rotation part of the matrix.
Parameters
- mSR [IN] 3x3 matrix - RMatrix3
Returns
- Return a new 4x4 matrix - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4()
2 matrix4_orgin.MakeIdentity()
3 matrix3_rotate_value = RLPy.RMatrix3( 1, 0, 0,
4 0, 1, 0,
5 0, 0, 1 )
6 matrix4_orgin.SetSR(matrix3_rotate_value)
GetSR (self)
Get scale and rotation part of the matrix.
Returns
- Return a 3x3 matrix - RMatrix3
1 matrix4_value = RLPy.RMatrix4( 1, 2, 1, 1,
2 1, 1,-1,-2,
3 1,-1,-1, 2,
4 1,-2, 1,-1
5 result = matrix4_value.GetSR()
6 row0 = result.GetRow(0)
7 print(row0[0])
8 print(row0[1])
9 print(row0[2])
SetTranslate (self, vTranslate)
Set translate of the matrix.
Parameters
- vTranslate [IN] Translate vector - RVector3
Returns
- New matrix with the specified translation - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4()
2 matrix4_orgin.MakeIdentity()
3 matrix4_orgin.SetTranslate(RLPy.RVector3( 1, 2, 3 ) )
GetTranslate (self)
Get translate of the matrix.
Returns
- Return a translate vector - RVector3
1 matrix4_orgin = RLPy.RMatrix4()
2 matrix4_orgin.MakeIdentity()
3 matrix4_orgin.SetTranslate(RLPy.RVector3( 1, 2, 3 ) )
4 result = matrix4_orgin.GetTranslate()
5
6 print(result[0] == 1)
7 print(result[1] == 2)
8 print(result[2] == 3)
AccuScale (self, rkScale)
Accumulate this 4x4 matrix with scale vector.
Parameters
- rkScale [IN] Scale vector - RVector3
Returns
- Accumulate of this 4x4 matrix with scale vector - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4()
2 matrix4_orgin.MakeIdentity()
3 matrix4_orgin.AccuScale(RLPy.RVector3( 2, 2, 2 ) )
4 matrix4_orgin.AccuScale(RLPy.RVector3( 3, 3, 3 ) )
5 result = matrix4_orgin.GetSR()
6 row0 = result.GetRow(0)
7 print(row0[0] == 2*3)
8 row1 = result.GetRow(1)
9 print(row1[1] == 2*3)
10 row2 = result.GetRow(2)
11 print(row2[2] == 2*3)
AccuRotate (self, rkRotate)
Accumulate this 4x4 matrix with rotation matrix.
Parameters
- rkRotate [IN] Rotation matrix - RMatrix3
Returns
- Accumulate this 4x4 matrix and rotation matrix - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4()
2 matrix4_orgin.MakeIdentity()
3 matrix3_orgin = RLPy.RMatrix3()
4 matrix3_orgin.FromAxisAngle( RLPy.RVector3( 0, 1, 0 ), 90 * RLPy.RMath.CONST_DEG_TO_RAD )
5 matrix4_orgin.AccuRotate(matrix3_orgin)
6 matrix4_orgin.AccuRotate(matrix3_orgin)
7 rotate = RLPy.RMatrix3()
8 matrix4_orgin.GetSimpleRotate( rotate )
9 row0 = rotate.GetRow(0)
10 print(row0[0])
11 print(row0[1])
12 print(row0[2])
AccuTranslate (self, rkTranslate)
Accumulate this 4x4 matrix with translate vector.
Parameters
- rkTranslate [IN] Translate vector - RVector3
Returns
- Accumulate of this 4x4 matrix and translation vector - RMatrix4
1 matrix4_orgin = RLPy.RMatrix4()
2 matrix4_orgin.MakeIdentity()
3 matrix4_orgin.AccuTranslate(RLPy.RVector3( 1, 2, 3 ) )
4 matrix4_orgin.AccuTranslate(RLPy.RVector3( 2, 2, 2 ) )
5 row3 = matrix4_orgin.GetRow(3)
6 print(row3[0] == 1+2)
7 print(row3[1] == 2+2)
8 print(row3[2] == 2+3)